

| Genre: | Educational (Ages 5-9) |
| Publisher: | Hodder & Stoughton |
| Cover Art Language: | English |
| Machine Compatibility: | BBC Model B, BBC Model B+, BBC Master 128, Acorn Electron |
| Release: | Professionally released on Cassette |
| Available For: | BBC/Electron |
| Compatible Emulators: | BeebEm (PC (Windows)) PcBBC (PC (MS-DOS)) Model B Emulator (PC (Windows)) Elkulator 1.0 (PC (Windows)) |
| Original Release Date: | 1st January 1984 |
| Original Release Price: | £9.95 |
| Market Valuation: | £2.50 (How Is This Calculated?) |
| Item Weight: | 64g |
| Box Type: | Cassette Single Plastic Clear |
| Author(s): | Bill Bailey & Brian Lienard |
Variant Items
There are 0 other items featuring this same game (that we know about!). Click any of them for their details.
Active Auctions
Closed Auctions
Buy It
Unfortunately no-one is currently selling this item.
Auction Price Watch
Worried you're being ripped off? Closing prices on eBay can help you decide what a reasonable price is for a particular item.
Full Instructions
Blurb
Every child needs to know their tables. With Merlin's help, every child will want to learn them too!
Most tables programs do little more than test and practise, but the clever design and exciting graphics of the Merlin programs really help children understand. In this pack, children discover the answers to tables problems in Merlin's Magic Tables and then apply their knowledge to finding factors in Merlin's Magic Ducks. Pack 2 takes children further with Merlin's Magic Skittles and Merlin's Magic Calculator.
The programs have been developed by Bill Bailey and Brian Lienard of London University, experts in both primary education and computing.
The Merlin Series
There are plenty of programs that test children on their tables. These programs are designed to help children understand and learn them.
There are four programs in this set: all on one disk or two separate pairs on tapes. Merlin's Magic Tables helps your child to find out what multiplications means, and to discover the answers to all the problems set. It's great fun, but there's no hurry and no pressure.
Merlin's Magic Ducks helps your child to apply the multiplication tables: to find out what division means, to see what a remainder looks like, to find factors and discover the prime numbers. (If you've forgotten, then join in! You'll both enjoy learning!)
Merlin's Magic Skittles is an entertaining action game designed to help children to learn the multiplication tables.
Merlin's Magic Calculator will give your child an idea of how a real calculator works. The magic lies in that it shows you what the quantities look like and the physical effects of multiplication, division, addition and subtraction.
But the computer cannot do everything! This booklet not only shows you how to get the most out of the programs, it also gives suggestions for other ways of helping your child to understand, apply and learn tables.
Merlin appears in all four programs and he is likely to appear in a lot more, so watch out for further programs from Hodder and Stoughton. The Estimation series of programs by the same authors are also designed to help numbers make sense to young children, and it is suggested that you use Butterflies And Putting from that series before using the Merlin programs.
Introduction
Most of us learned our tables by reciting them out loud. This is a very effective way of fixing them in the memory. But very often children who learn tables in this way, don't really understand what they are all about. They might 'know their tables', but they don't know what to do with them!
If you say '5 x 7' or '7 x 5', the child can tell you the answer, 35. But if you say 'I have 5 cups of tea every day. How many cups of tea do I drink in a week?', the answer takes longer to appear; if they can do it all. It's no use knowing your tables if you don't know when to use them.
Modern primary schools, quite rightly, stress the need to understand arithmetic and mathematics; to know why numbers behave the way they do. Modern methods sometimes disturb parents, because they feel children don't have the 'facts at their fingertips'. This worry should not arise, because most educators believe that knowing the facts is still important. What we really need is both knowledge and understanding. Understanding without 'automatic knowledge' slows down arithmetic. 'Automatic knowledge' without understanding delays real progress in mathematics. If children cannot apply their knowledge, it is useless. Merlin intends to teach your child both to understand the tables and to know them.
How does this work? First of all, the programs are visual. Children can always see what is going on. In Merlin's Magic Tables they see '7 x 5' as 7 lots of 5 magic objects. (Real magic! They are a different set every time you use the program!) Whatever number is put in (up to 100) will be shown as objects. So children can see if they have another go, until they reach the right answer. Children are not stupid. If they can see what is happening, then they behave intelligently.
Consider what happens in programs where children see a problems which says '7 x 6' using only the written numbers on the screen. They either know the answer or they don't! If they don't the screen is not going to help them to find out! The Merlin programs do help them. All the time.
The same idea is applied in Merlin's Magic Ducks. If the child succeeds in finding a factor, the last duck lays an egg in the tray. If the child is wrong, then the remainder is always obvious. The number of ducks on the screen is the number the child is dealing with. Nothing is hidden. Everything is visible.
Merlin's Magic Skittles also uses 'visual numbers'. The game can be played in two ways. If the child needs to take time to work out the answers, then this is allowed. But the game is designed to be played fast. If the other programs have been used a lot, then this program should be used as a high speed game to get the tables 'at the fingertips'. Merlin can only show your results if the game is played in this way.
Merlin's Magic Calculator is not a game. It doesn't set problems either. It is meant to be a tool. If you want to know what 25 + 47 is, it will show you. In three different ways. You can see what the answer is as a sum:
| 25 + 47 = 72 |
You can see how the window on a pocket calculator shows the numbers:
| 2 ... 25 ... (+) ... 4 ... 47 ... (=)... 72 |
And particularly, you can see:
| what 25 objects look like, | what 47 objects look like, | and what they look like when added together to create 72 objects. |
The calculator also handles subtraction, multiplication and division.
In each program there are several options for the parent. This means that you can control the way the child plays. All children are different, so we have made it possible for you toadjust the programs to suit your child. It is very important that you know how to use the options. So make sure you are familiar with them before you begin using the programs with children.
Merlin's Magic Tables
This program is designed to show children what multiplication means. Multiplication can be seen as addition. Take 3 for example. Add another 3 and you get 6, add another and you get 9 and so on. When you have done this 10 times you have got 30. The 3 times table is therefore a list of all the answers to this process of repeated addition. Committing it to memory can be very useful, but first it is important to understand what is going on. This program illustrates the process by showing the children pictures of objects, set out in the form of the table.
This means that even if they can only count, they can work out the answer to any problem set. If they mis-count and are not quite right, it doesn't matter because they can see how far out they are and correct themselves.
Which way around should we set the problems? There are two ways of looking at the following expression:
7 x 3 = 21
It could be seen as 'seven times three' meaning 'seven lots of three' or 'take three seven times'. Like this:
3 + 3 + 3 + 3 + 3 + 3 + 3
Another way of looking at it is to read it as 'seven multiplied by three'. This would be understood as a set of seven items repeated three times. Like this:
7 + 7 + 7
How did you learn to recite your tables? Did you say: 'One three is three (1 x 3 = 3), two threes are six (2 x 3 = 6), three times are nine (3 x 3 = 9)', etc? Or did you say: 'Three ones are three (3 x 1 = 3), three twos are six (3 x 2 = 6), three threes are nine (3 x 3 = 9)', etc? In one sense it doesn't matter whether you say seven threes or three sevens, because either way around the answer is 21, but if your child is learning it one way at school and another way at home, you are in danger of producing confusion. We have therefore built-in an option (see page 10), so that you can choose to have the programs presented either way. In order to choose the correct option, it is important to find out which way the table is set out in the maths scheme in your child's school.
Using The Program
Once you have loaded the program you will be asked up to three questions:
Do you want Merlin to choose the table?
If you answer Y for Yes, then you will be asked the next question:
Do you want a hard table?
If you answer Y for Yes, then Merlin will choose randomly from the 3, 4, 6, 7 or 9 times tables. If you answer N for No, he will choose from the 2, 5 or 10 times tables (but see Options on page 11).
Suppose you answer N for No, when asked if you want Merlin to choose the table. This means that you can choose it yourself. You then simply type in the number of the table you want to work on.
The last question to answer is:
Do you want the table jumbled?
If you answer Y for Yes, then the problems will appear randomly. If you answer N for No, Merlin will take you through the table in order, from 1 to 10.
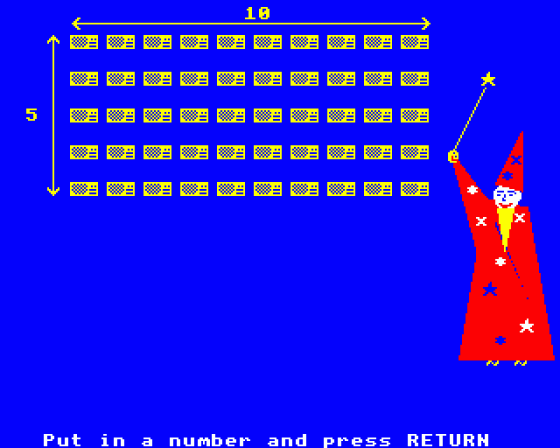
Merlin will now present a problem. Let's suppose the 7 times table has been chosen, and we have decided to do it jumbled. The first problem might be 6 x 7. You will see a row of 7 objects repeated 6 times, and a question will appear at the bottom of the screen asking you to type in the answer. If you type in the correct answer, 42, then Merlin lets you know you are right by flashing stars with a sound effect.
However, if you are wrong, he doesn't justtell you so and move on to the next problem. He shows you what your answer looks like. Suppose you typed in 36 instead of 42. One at a time the yellow objects change to red, until 36 of them have changed, and they are accompanied by musical tones which ascend in groups of 7. The rest of the objects are left yellow, and your number, 36, is printed under the last red object. So you can see that 36 is too small.
Children need time to think when learning, and shouldn't be put under pressure. What is more, if they are wrong and can see how they are wrong, they ought to be allowed to use this information for another try. Merlin lets them take as long as they need and to have as many goes as they like until they are successful!
When a wrong answer is put in, Merlin prints the written form of the table alongside the objects on the screen, so that children can see how the table builds up. They can then count on from their wrong answer to find the right number and put it in. The rest of the objects then change colour one at a time to reach the right answer.
What happens if the child puts in a number that is too big? Provided it is not bigger than 100, Merlin colours all the yellow objects red, and then continues to put red outline objects on the screen up to the child's number. At the same time the table is printed on the screen as it builds up. Suppose the child put the answer 84 for 7 x 9. The 63 objects on the screen would turn red. Then outline objects would complete the table up to 70, 7 x 10.
After that outline objects appear down the edge of the screen, accompanied by beeps, but still in groups of 7, up to the child's guess of 84.
Options
One of the most important features of the Merlin programs is their flexibility. The program can be run as described above, but we have made it possible to tailor it easily to your own child and situation.
To get to the options, you must press the key marked @ (next to P) while the next page of the program, Merlin's Magic Tables, is on the screen. You will then be asked a series of questions:
| 1. | Pause (value)? When the objects change from yellow to red there is a slight delay between each row. This pause can be changed so that children have time between rows to see what is happening and could practise saying their table as the numbers appear. The value is in hundredths of a second. So to put one second pause between rows, type 100; for half a second, type 50; for one and a half seconds, type 150, and so on. Type in the pause you want and press RETURN. |
| 2. | T. No. Right? This is short for 'Table Number Right?'. If you type Y for Yes, the table number will be presented as the number to the right in each problem: 1 x 3 = 3, 2 x 3 = 6, etc. If you say N for No, the table will appear as 3 x 1 = 3, 3 x 2 = 6, etc. To decide which way to respond you should ideally find out which way round the tables are set out in the maths scheme used in your child's school. |
| 3. | Symbols? If you just want the problems presented as pictures, with no numbers cluttering up the screen, you answer N for No. |
| 4. | Sound? If you don't want the sound effects, you can switch themoff. Just type N for No. |
| 5. | Colour? If you have a black and white TV or monitor, type N to select the 'no colour' version. |
| 6. | Scores? Some people prefer not to give children scores for their efforts. If you don't want scores reported at the end, type N. |
| 7. | Classify? Which tables are 'easy' and which tables are 'hard' will not be the same for everybody? We have decided to call 2,5 and 10 'easy', and 3, 4, 6, 7, 8 and 9 'hard'. We have also decided to make the 1 times table unavailable. But you are not stuck with our categories. If you type Y for Yes, then you will see: Classify? Press Y for Yes, N for No: Y 1 x (E/H/U)? This is asking you if you want Merlin to treat the 1 times table as Easy, Hard or Unavailable. So you type E or H or U depending on what you decide. You are then presented with the next table: 2 x (E/H/U)? and so on. You can always return to the title page by pressing the BREAK key. |
Making The Most Of The Program
This program is really meant for children who do not yet know all their tables. If you are using it with children who are still in the infant school age range then it is probably best to start with the 2 or 10 times table, and to let them work through these in the ordered version. (You select this by saying no when asked if you want the table jumbled.) It should be possible to show the child how to count on from the last problem to get to the new one. Suppose you have just done: 2 X 2 = 4. The next problem will be 3 X 2. You could say: 'There were 4 objects last time, now we have 2 more, so it has to be 4, 5, 6,' pointing to the objects on the screen as you count them. Encourage the child to find the number 6 on the keyboard and to press RETURN. In this way you can work your way through the table with the child. The next step might be to let the child try the table without your help. If they quickly get the hang of it and can do the table in order, then you can get them to use the jumbled version.
The 10 times table is particularly easy to learn, since it involves only adding a 0 to the question. However, because in this program the child can see what 60 objects look like for 6 X 10, a feel for number is being built up in a way which is less easily grasped using numbers alone.
The 5 times table is also one of the easiest to learn. The repeated pattern of fives and zeros which alternate as the rows build up and can be spotted by children. You may need to draw their attention to this.
The 3 and 4 times tables are probably the next easiest to deal with since the numbers are small.
The 6, 7, 8 and 9 times tables are the ones tat children usually have the most difficulty in learning. However, the answers to the 9 times table form an easy pattern to notice:
9, 18, 27, 36, 45, 54, 63, 72, 81, 90
When you ask them, children usually notice that the tens go up in ones:
1, 2, 3, 4, 5, 6, 7, 8, 9
They also notice that the units go down in ones:
9, 8, 7, 6, 5, 4, 3, 2, 1, 0
What they may not notice however is that if the digits are added together they always make 9!
Take 36 for instance. If we add 3 + 6 we get nine, 54 gives 5 + 4 = 9, 81 gives 8 + 1 = 9. So all the answes to the 9 times table add up to 9. If you know this you can tell straight away that 9 X 7 isn't 42!
Remembering that the tens go up in ones, then two nines must start with 1, seven times nine must start with 6, nine times nine with 8 and so on. In this way you can always work out the first digit, and then all you need to do is add the number which makes a pair which add up to 9.
Another way of finding the answers to the 9 times table is to use the fingers of both hands. If you open out both hands in front of you with the palms towards you, you will find an instant '9 times table calculator'! You want to know what four nines are? Just bend down your fourth finger. The remaining figures provide you with the necessary digits. There are 3 finders up to the position occupied by the fourth finger. This gives you the tens. After the position of your fourth finger there are six fingers. These tell you the units. So, 4 X 9 is 3 tens and 6 units, 36. Similarly, if you want to know what 8 nines are, you'll find 7 fingers on the left of number 8, and 2 on the right of it. Answer = 72!

Children should always be encouraged to notice patterns in numbers. Patterns help to reduce the load on memory. When there is no obvious pattern it is still possible to arrive at correct answers if the child understands what is happening. Whate are nine eights you ask? The child may not know. However, if they know that ten eights are eighty, and can see multiplication as being repeated addition, then they only need to take eight off eighty to find that nine eights are 72.
Parents and teachers should be pleased to see children performing like this because it shows a level of understanding. But in the end we need to know our tables 'quickfire'. The third program in this series Merlin's Magic Skittles is specifically designed to test children's ability to do them in this way, but there is no reason why committing them to memory shouldn't accompany use of this first program.
Most of us learned our tables by reciting them out loud a large number of times, perhaps in chorus with the rest of our school class. Those of us who still remember them are therefore convinced that this is the best method! For a child, trying to commit a list of ten 'number facts' to memory is probably not very exciting. Even though computer tables games can be an incentive, mastering a list of ten may still not be easy.
One approach is to break it down into smaller steps. Ask your child to learn only the first three. Let's take the eight times table as an example. To remember 8, 16 and 24 should be easy enough. 'Test' the child by jumbling them. 'Two eights? ... One eight? ... Three eights?' etc. They should find this easy. Now ask them to learn the next three, 32, 40, 48. Test them on these separately and ten when they know them, put all six together, and then jumble them. At this point over half the table is learned. Ten eights is easy, so there are only three more things to learn. When the child seems to know them let them practise with the program, first of all in order and then jumbled. They can later use the Magic Skittles program to see how fast they are and to increase their speed.
These are just tricks to aid the memory. Using them with the computer's ability to show the child what the actual quantities look like should give them real meaning. However, it is very important that children are given every opportunity to see multiplication in use and to find examples in their own environment.
Multiplication Games
Look around you. If you have the 2 times table in mind, ask the child to find things which come in twos. Some obvious ones are: shoes, socks, gloves, hands, feet, eyes, bicycle wheels. You could invent some problems based on these. How many hands are there in our family? How many socks have you got? Children don't always find it easy to distinguish the number of groups from the number of objects. This kind of experience should help. Talking about number is important, but do so when the child can see clearly what the words refer to. For example 'How many pairs of red socks have we got?' when they are folded in pairs. And then, 'So, how many red socks have we got?' as you open the pairs out to reveal the socks individually and count them.
Two pence coins could be used: 'How much is four two pence pieces?' Put out four two pence pieces and then get the child to replace each one with two pennies. Count the pennies.
This might also be an opportunity to teach the child to count in twos. The way to do it is to count in ones, whispering alternate numbers: one, TWO, three, FOUR, five, SIX, seven, EIGHT, nine, TEN. After a few goes up to ten ask the child to say the whispered ones so quietly you can't hear them. Then challenge them to go up to twenty. Once they've made it, they know the answers to the 2 times table. Transfer this to the computer program. Let them see if they can get the 2 times table right with Merlin.
You should be able to find examples of the other tables, or be able to create them. Using matchsticks you could make triangles and ask how many matches are needed to make five triangles, etc. Turn the game round and ask the child to create triangles and see how many matches are needed to make a certain number. In this way you could practise the 3 times tables.

Making squares with the matches is also a way of practising the four times table. Looking at how many wheels on a number of toy cars would be another way.
The minutes round the clock face are an example of the 5 times table in use.
Empty half-dozen egg boxes would give you an example of the 6 times table.
The 7 time tables is found on the calendar: 'How many days are there in 6 weeks?' etc.
The links between the tables should be pointed out. Parts of the 4 times table appear in the 8 times table. So does some of the 2 times table. Parts of the 3 times table appear in the 6 times table and in the 9 times table.
One pencil and paper exercise would be to write down a number, and go on adding that number on to it. Suppose we start with 3. The next number to write down is 3 + 3, i.e. 6. The next number is 6 + 3, then next is 9 + 3, and so on. This of course gives the sequence 3, 6, 9, 12, 15, 18, 21, 24, 27, 30, which is the 3 times table. Starting with 4 and adding on fours gies you the 4 times table. (Many children don't want to stop after ten numbers have been written down, and find it a challenge to keep going up to 100!) It oftens happens that a mistake early in the sequence throws the rest out. A way to check this would be to compare the answers with the same table set by Merlin on the computer.
Mathematicians say that multiplication is commutative. You may never have heard the word before but you know what it means! It means that if you know that 6 X 7 is 42, then you ought to know that 7 X 6 is 42! In other words the answer is the same whichever way round you put the two numbers. (Is this true if you are doing subtraction?)
Once children are aware of this it considerably reduces the number of facts to be learned considerably. If you can't remember what six eights are, you might be able to remember what eight sixes are! This can easily be pointed out to children when they are looking at one of the displays in the program.
There is no substitute for plenty of experience and discussion with real objects, and real problems at first hand, but the computer does have a role to play. It can show children the objects. The problem of 'How many crowns?' on the screen is just as real as 'How many wheels?' on the toy cars.
Children can get fascinated by the patterns that show up in numbers, and enjoy playing with them even if they don't have an immediate purpose in serious calculation. Squared paper can provide lots of fun with numbers. A 10 by 10 square can be used to discover patterns. If the child is asked to colour every third square and put the numbers in until they have coloured in ten squares then they will have the 3 times table. They could then colour in every sixth square and see what the pattern looks like. Some numbers will appear in both patterns. Why?

The 10 by 10 square will give a different pattern for each table. You could cut out squares other than a 10 by 10 square. How many little squares appear in the 7 x 7 square? In this way children could be encouraged to explore sqaure numbers: 2 x 2, 3 x 3, 4 x 4, 5 x 5, 6 x 6, 7 x 7, etc, i.e.
4, 9, 16, 25, 36, 49, 64, 81, 100.

Notice that when these numbers appear in their respective tables in the program the layout of the objects does actually form a square.
Rectangles cut from squared paper can be used to illustrate number patterns.

A 10 by 10 square can also be turned into a 'multiplication square'. All you do is write the numbers 1 to 10 across the top and down the side. You could show how the square numbers fall along the diagonal of this square, and also that all the answers one way repeat themselves when you ask the question the other way round.

Yet another way to illustrate tables and to look for pattens in number is to use what is known as the 'number line'. All you need to do is to write down the numbers, say up to 100, along a strip of paper or card. You could use small sticky labels from a stationer's and write the numbers on these. Make sure they are evenly spaced. You can use it to show for example that 12 is 3 x 4, and also 4 x 3; and 2 x 6.

If the child is dealing with real objects, and that includes those on the screen, then it is always possible to find the total number in a table by counting them. The fact that there is the same number in each row means that the next thing to understand is that repeated addition can give the answer. The final thing to know is that multiplication tables are an even quicker way.
Look for examples of regular tables of things around you: tiles on walls, floors or ceiling; panes in windows; handles on drawers. Lego bricks are an endless source of tables for counting and could be used to set problems for applying tables knowledge.
Merlin's Magic Ducks
This program is designed to help children to think about numbers and to make use of the knowledge of multiplication gained from Merlin's Magic Tables.
They have to apply this knowledge to finding the factors of numbers. Take the number 12 for example. They may know that 12 = 2 x 6. This can be turned round to show that 12 = 6 x 2. 12 can also be made from 4 lots of 3, or 3 lots of 4. If we write down all the combinations which multiply to make 12, we get:
| 12 x 1 | = | 12 |
| 1 x 12 | = | 12 |
| 2 x 6 | = | 12 |
| 6 x 2 | = | 12 |
| 4 x 3 | = | 12 |
| 3 x 4 | = | 12 |
If we make a list of all the numbers we have used, here they are:
1, 2, 3, 4, 6, 12
These numbers are called the factors of twelve. If we divide 12 by any of them we find that there is no remainder. They all go nicely into 12 without anything left over. If we were to divide 12 by 5, we would have 2 left over, so 5 is not a factor of twelve. 7 won't go into 12 without a remainder, and the same applies to 8, 9, 10, 11.
What happens with a number like 13? We get a remainder if we try to divide by 2 (or 3, 4, 5, 6, 7, 8, 9, 10, 11 or 12). So the only numbers which will multiply together to make 13 are:
| 1 x 13 | = | 13 |
| and 13 X 1 | = | 13 |
This means that 13 is a prime number. Prime numbers are numbers with only two factors. 1 and themselves.
Using The Program
Merlin's Magic Ducks allows children to discover the factors of a number. So first of all a number has to be chosen. Once you have loaded the program you will be asked:
Do you want Merlin to choose the number?
Press Y for Yes, N for No.
If you say yes, then Merlin will pick a number between 1 and 100. If you say no, then you can choose the number.
Let's suppose that 36 has been picked. You will then see 36 white ducks all facing left, in 3 rows of 10 and a shorter row of 6. Underneath the bottom row of ducks is an 'egg tray'. This looks rather like a ruler because the sections of 10 are in alternate colours. This means that it can act as a 'number line'. The first 'slot' represents the number 'one' and the last slot 'fifty'. At the top right of the screen you are reminded how many ducks there are:
36
Ducks
At the bottom of the screen you are invited to 'Put in a number and press RETURN'.
Suppose we put in 4. We will now see whether 4 is a factor of 36. One at a time the first 4 magic ducks will turn round to face right, and change colour from white to yellow. A tone will sound as each one changes. After a slight pause the next 4 magic ducks will turn to face right and change from white to blue. This process will continue until we get to the last duck. When this one turns round it lays an egg which is then funnelled into the egg tray. When it arrives it goes into the fourth slot in the egg tray and this is then labelled with the number 4. In this way children can see that 4 goes nicely into 36 and is a factor of 36.
You can go on putting in numbers in this way. Sets of yellow and blue ducks appear until no more will fit. If the number put in is not a factor, then there will be a remainder, and the last duck, or ducks, will still be white and facing left. If this happens then no egg is laid. After trying several numbers the child should be able to see which ones were factors by looking at the egg tray.
Also at the bottom of the screen it says:
P -> Prime, A -> All found, H -> Help:
When you think that there are no more factors to be found in the number, press A. If you are right, then Merlin will let you know that you have found them all. However, if there are more to be found, you will get the message: 'You have not found them all yet' and you can try another number.
If you are really stuck and can't think of any more, then press H for Help. Merlin won't give you the answer, but he'll give you a clue, by putting a series of 5 question marks (?????) over the part of the egg tray where you can find another factor.
Of course some numbers don't have factors other than themselves and 1, so if you think that the number you are working on is one of these, then you can type P, to tell Merlin that you think the number is a prime number. If it is, then you have finished. If it isn't, you must think again.
Numbers between 50 and 100 all have themselves as factors, but these can't be positioned on the number line because it only goes up to 50. In this case, Merlin very cleverly uses his magic wand to catch the egg and put it beside the number itself, at the top right of the screen.
Once the task is finished, and all the factors have been found, and after the magic end, the display stays on the screen so that you can talk to the child about the patterns of the numbers in the egg tray.
The program therefore enables children to discover the factors of any number up to 100, and to decide which numbers from 1 to 100 are prime numbers. If they know their multiplication tables then the task should be easier. If they don't really understand multiplication, then this game, which demonstrates one way of doing division, should help that understanding to develop.
Options
Like Merlin's Magic Tables, this program has built-in flexibility. You can get to the options by pressing the @ key when the title page, Merlin's Magic Ducks, is on the screen. You will then be asked a series of questions:
| 1. | Pause (value)? After each set of coloured ducks appears there is a slight pause. If you want the process to run more slowly for the child to take it in, you can increase the time delay between the sets. The time value is in hundredths of a second. So to put one second pause between sets, type 100; for half a second, type 50; for one and a half seconds, type 150 and so on. Type in the pause you want and press RETURN. |
| 2. | High (3-100) Large numbers might be daunting for very young children. If, for example, you only wanted to find factors of numbers up to 20, simply type 20 and press RETURN. |
| 3. | T. No. Right? This is short for 'Table Number Right?'. If you type Y for Yes, the table number will be presented as the number to the right in each problem: 1 X 3 = 3, 2 X 3 = 6, etc. If you say N for No, the table will appear as 3 X 1 = 3, 3 X 2 = 6, etc. To decide which way to respond, you should ideally find out which way round the tables are set out in the maths scheme used in your child's school. |
| 4. | Sound? Type N to switch off the sound effects. |
| 5. | Colour? If you have a black and white TV or monitor then type N to select the 'no colour' version. |
Making The Most Of The Program
If we think of multiplication as 'repeated addition' we can think of division as 'repeated subtraction'.
What does 12 ÷ 3 = 4 mean? We can look at it in two ways. One is to say 'How many threes are there in twelve?' This means how many groups of 3 do I need to make 12. Or, how many times can I take 3 away from 12 before I have none left, i.e. repeated subtraction.
Another way is to see division as a sharing problem. If I share 12 into 3 equal parts, how many in each part? Sharing 12 into 3 parts is also a way of asking 'What is a third of twelve?' In other words, relating it to fractions.
The program Merlin's Magic Ducks only deals with one of these interpretations. It treats division as repeated subtraction. If you are shown 27 ducks and you guess that 3 is a factor, it shows you how many times 3 will fit into 27.
What we are really doing is showing the child that factors and multiplication tables are linked. If the number 24 appears in a multiplication table then the table it is in gives you a factor. For example, the 8 times table has 24 in it. So 8 is a factor. The 6 times table has 24 in it, so 6 is a factor. And so on. One of the things that this program should help to bring out is that multiplication is commutative. If 6 goes into 24 without a remainder, then 6 is a factor. If we now notice that 6 went into 24 four times, then 4 is also a factor.
The term factor is not usually introduced until well into the junior school, but the idea is simple enough. Children as young as five can play this game once you have explained how it works. E.g. 'What you have to do is guess a number and see if it fits nicely, so that there are no white ducks left.'
You would probably be best advised to put in the first number rather than let Merlin choose it. Use a fairly small number with young children, but choose one that has several factors for them to find. 12 is probably a good example to start with. They can try 1, 2, 3 and 4 as factors and find that they all fit, but when they try 5, then they will see that you mean by a remainder. It doesn't take long for them to get the idea of the game and then they can explore on their own.
Prime Numbers
Many people are under the impression that prime numbers are numbers which don't have any factors. Mathmatically a prime number is a number which has only two factors, itself and one. (In this definition 1 is not a prime number.) For example, if you try to find numbers which will fit nicely into 13 without any remainder, you will only find that 13 and I fit. so this defines 13 as a prime number.
If Merlin chooses a number and you think it is a prime number the program allows you to say so without having to go through all the possible factors. You could challenge children to find all the numbers up to 10, which are prime. Then up to 20, 50, 100. It is quite a challenge.
A mathematician called Eratosthenes, in the third century discovered a quick way of doing this. It became known as the Sieve of Eratosthenes. We can illustrate it with the 10 by 10 square.

| 1. | First fill in all the even numbers. These can't be prime because they will all divide by 2. |
| 2. | Now go through and fill in all the numbers which make up the 3 times table. Carry on filling in 33, 36, 39, 41, etc. up to 99. |
| 3. | Now fill in all the numbers in the 5 times table. Carry on filling in all the multiples of 5 until you get to 100. |
| 4. | Finally go through and fill in numbers in the 7 times table, and carry on up to 98. |
| 5. | Fill in number 1. |
The numbers which are left are the prime numbers up to 100. To sum up: To test for a prime number you only need to divide it by 2, 3, 5 and 7. If these don't fit them then no other numbers will, so it's prime.
Patterns
If you have a lot of counters, you could show how the factors of a number can be used to make different rectangles. The child could also draw patterns like the ones of page 22 on square paper.
The way that the array of ducks is shown on the screen means that you can point out how numbers are composed of tens and units. Counting the complete rows of ten tells you the first digit of the number, counting the units gives you the second. Children need to understand this very important idea, whic is called place value.
Although the layout of the ducks on the screen is as tens and units, interesting patterns do appear when the ducks change roung and become alternate sets of yellow and blue. You can give children more time to think between sets by increasing the pause using the options. The final display stays on the screen for as long as you want. This means that you can discuss the patterns with the child. (Pressing the SPACE bar clears the pattern.) Fractions can be considered by looking at the finished display of a successfully found factor. For example, suppose we were looking for factors of 60, and had found 15 was a factor. You could point out how 4 of them fit, and how 2 of them fit into half the display. In this way, 15 can be seen as a quarter of 60. If we now look at the pattern that emerges when we try 12 as a factor, then we can show that 12 is one fifth of 60. Turning it round and trying 5, we can show how 5 is one twelfth of 60.
There are also patterns to detect in the numbers under the eggs in the egg tray. If you have tried 3 as a factor of 24, the bottom left of the screen will go through the three times table as the sets of coloured ducks build up. It will finally say '8 X 3 = 24'.
This should tell the child that 3 is a factor and it fits 8 times, so 8 must also be a factor, and it fits 3 times. In this way for every factor you can find there should be another one which pairs with it. e.g.

Which numbers don't have an even number of factors? Why?
When all the factors of a number have been found, it is possible to draw factor trees. For example, let's suppose we have found the factors of 24. We can build up several different trees by showing how 24 can be broken down into its factors.
When you reach the bottom row, you have numbers which can't be broken down any further. These numbers must be prime numbers. They are therefore called the prime factors. In the example here, the factors of 24 are 1, 2, 3, 4, 6, 8, 12. The prime factors of 24 are 2 and 3. So another challenge is to see what are the prime factors of a given number.
Screen Designers
The following utilities are also available to allow you to edit the supplied screens of this game:
Cheats
Download
A digital version of this item can be downloaded right here at Everygamegoing (All our downloads are in .zip format).
| Download | What It Contains |
|---|---|
| A digital version of Merlin Teaches Tables 1 suitable for BeebEm (PC (Windows)), PcBBC (PC (MS-DOS)), Model B Emulator (PC (Windows)), Elkulator 1.0 (PC (Windows)) |
Report A Problem
We thank you from the bottom of our hearts if you report something wrong on our site. It's the only way we can fix any problems!
You are not currently logged in so your report will be anonymous.
Add Note
Release Country
Change the country to update it. Click outside of this pop-up to cancel.
Scan Of Selected Article
If you auction an item, it will no longer show in the regular shop section of the site.







